Purnachandra Saha and R. S. Jangid1 Department of Civil Engineering Indian Institute of Technology Bombay, Powai, Mumbai-400 076, India.
Download Citation:
|
Download PDF
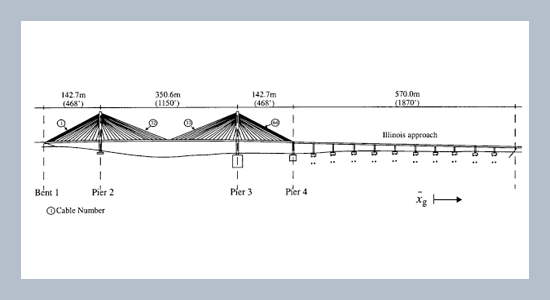
Earthquake response of benchmark cable-stayed bridge with different isolation systems is investigated. The selected isolation system consists of high damping rubber bearing (HDRB), lead-rubber bearing (LRB), friction pendulum system (FPS) and resilient-friction base isolator (R-FBI). Considering the phase-I benchmark problem, the ground acceleration is only applied in the longitudinal direction acting simultaneously at all supports. The seismic response of the benchmark bridge is obtained by solving the governing equations of motion of bridge by Newmark’s step-by-step integration method. A comparative performance study among the selected isolators for seismic response control of bridge is carried out. A parametric study for investigating the effectiveness is also performed with variation of important isolator parameters. Varying the different parameters of the isolators, evaluation criteria of the benchmark cable stayed bridge problem are found out. Significant reduction in base shear, overturning moment and other responses are observed by using the control systems by seismic isolator. Comparing the evaluation criteria of the benchmark problem, it is observed that the performance of LRB and R-FBI are better than that of the HDRB and FPS. Further, increase in the bearing damping ratio reduces both device displacement and base shear for HDRB and LRB. The effects of device isolation period on structure depend on the isolator as well as the type of selected input earthquake motion.ABSTRACT
Keywords:
Benchmark cable-stayed bridge; HDRB; LRB; FPS; R-FBI; seismic response; base isolation
Share this article with your colleagues
REFERENCES
ARTICLE INFORMATION
Accepted:
2008-12-27
Available Online:
2008-11-01
Saha, P., Jangid, R.S. 2008. Comparative performance of isolation systems for benchmark Cable-stayed bridge. International Journal of Applied Science and Engineering, 6, 111–139.https://doi.org/10.6703/IJASE.2008.6(2).111
Cite this article: