Deepak Kumar* and S. B. Singh Department of Mathematics, Statistics and Computer Science, G. B. Pant University of Agriculture and Technology, Pantnagar
Download Citation:
|
Download PDF
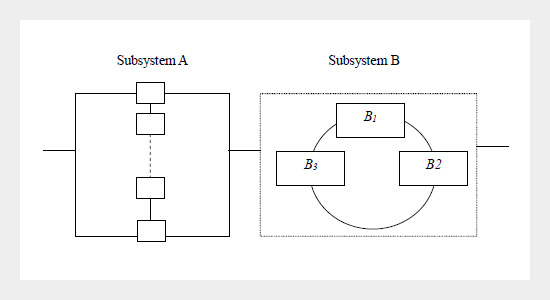
Present paper discusses the reliability analysis of a complex system which consists of two repairable subsystems namely A and B connected in series. Subsystem A is a k-out of -n: G system and subsystem B is a circular consecutive 2-out of -3: F system. In this study an inspection shop has been taken into consideration along with a special type of delay viz. reboot delay. By employing supplementary variable technique, Laplace transformation and Gumbel-Hougaard family of copula various transition state probabilities, reliability, availability, M.T.T.F., cost analysis, sensitivity analysis and steady state behaviour of the system have been obtained. At the end some special cases of the system have been taken and important results have been derived as particular cases.ABSTRACT
Keywords:
Reliability; availability; mean time to failure; sensitivity; reboot delay; complex system; gumbel-hougaard family of copula
Share this article with your colleagues
[1] Chung, W. K. 1993. Reliability analysis of a K-out-of-N redundant system with the presence of chance with multiple critical errors. Microelectronics and Reliability, 33, 3: 331-334.REFERENCES
[2] Coit, D. W. and Liu, J. 2000. System reliability optimization with k-out-of-n subsystems. International Journal of Reliability, Quality and Safety Engineering, 7, 2: 129-142.
[3] Goel, L. R. and Gupta, P. 1984. Stochastic analysis of a two unit parallel system with partial and catastrophic failures and preventive maintenance. Microelectronics and Reliability, 24, 3: 881-883.
[4] Gupta, R. and Bhardwaj, P. 2013. A two-unit standby system with two operative modes of the units and preparation time for repair. Journal of Reliability and Statistical Studies, 6, 1: 87-100.
[5] Hassett, T. F., Dietrich, D. L., and Szidarovszky, F. 1995. Time varying failure rates in the availability and reliability analysis of repairable systems. IEEE Transactions on Reliability, 44, 1: 155-160.
[6] Ke, J. C., Lee, S. L., and Ko, M. Y. 2011. Two-unit redundant system with detection delay and imperfect coverage: cofidence interval estimation. Quality Technology & Quantitative Management, 8, 1: 1-14.
[7] Ke, J. B., Chen, J. W., and Wang, K. H. 2011. Reliability measures of a repairable system with standby switching failures and reboot delay. Quality Technology & Quantitative Management, 8, 1: 15-26.
[8] Moustafa, M. S. 2001. Reliability analysis of systems with time varying failure rates. Economic Quality Control, 16, 2: 183-191.
[9] Ram, M. and Singh, S. B. 2008. Availability and cost analysis of a parallel redundant complex system with two types of failure under preemptive-resume repair discipline using Gumbel-Hougaard family of copula in repair. International Journal of Reliability, Quality & Safety Engineering, 15, 4: 341-365.
[10] Ram, M. and Singh, S. B. 2010. Analysis of a complex system with common cause failure and two types of repair facilities with different distributions in failure. International Journal of Reliability and Safety, 4, 4: 381-392.
[11] Ram, M. and Singh, S. B. 2010. Availability, MTTF and cost analysis of complex system under preemptive-repeat repair discipline using Gumbel-Hougaard family copula. International Journal of Quality & Reliability Management, 27, 5: 576-595.
[12] Trivedi, K. S. 2002. “Probability and Statistics with Reliability, Queuing and Computer Science Applications”. 2nd Edition. John Wiley & Sons. New York.
[13] Yam, R. C. M., Zuo, M. J., and Zhang, Y. L. 2003. A method for evaluation of reliability indices for repairable circular consecutive-k-out-of-n: F systems. Reliability Engineering and System Safety, 79: 1-9.
ARTICLE INFORMATION
Received:
2013-04-08
Revised:
2013-08-13
Accepted:
2013-08-27
Available Online:
2013-12-01
Kumar, D., Singh, S.B. 2013. Reliability analysis of embedded system with different modes of failure emphasizing reboot delay. International Journal of Applied Science and Engineering, 11, 449–470. https://doi.org/10.6703/IJASE.2013.11(4).449
Cite this article: