Tzu-Hsin Liu1, Chih-Meng Wang2, Yih-Bey Lin1, Fu-Min Chang1* 1 Department of Finance, Chaoyang University of Technology, Taiwan
2 Ph.D. Program of Business Administration in Industrial Development, Chaoyang University of Technology, Taichung, Taiwan
Download Citation:
|
Download PDF
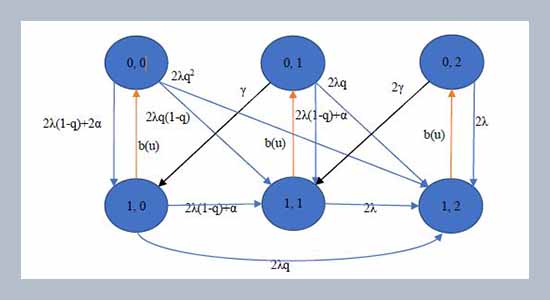
A robust power supply system with high availability and acceptable cost/benefit is essential for many service systems, such as communication networks and product manufacturing processes. This investigation is concerned with the evaluation of the cost-benefit of a standby retrial power supply system incorporating standby switching failure and general repair times, which is the first work on the comparative analysis of retrial availability systems incorporating switching failure and general repair times. Four different standby power supply retrial configurations are included and each configuration consists of a different number of primary and standby generators. The investigated system assumes that the time-to-failure and the time-to-repair of the primary and standby generators obey the exponential and general distributions, respectively. We also take that the switching over standbys may be failed into account. By using the supplementary variable technique, the explicit expressions of the steady-state availability for each configuration are derived. A comparative analysis of the availability and the cost-benefit ratio among four retrial systems is presented. We also rank the configurations based on the steady-state availability and cost-benefit ratio for two repair time distributions, Weibull and lognormal. The calculated numerical results can provide managers with decision reference for stable power supply system and cost reduction.ABSTRACT
Keywords:
Retrial queue, Availability, Switching failure, Cost-benefit, Supplementary variable.
Share this article with your colleagues
REFERENCES
ARTICLE INFORMATION
Received:
2021-06-23
Revised:
2021-07-28
Accepted:
2021-10-01
Available Online:
2021-12-01
Liu, T.-H., Wang, C.-M., Lin, Y.-B., Chang, F.-M. 2021. Evaluation of the cost-benefit of standby retrial systems incorporating switching failure and general repair times. International Journal of Applied Science and Engineering, 18, 2021213. https://doi.org/10.6703/IJASE.202112_18(6).004
Cite this article:
Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.