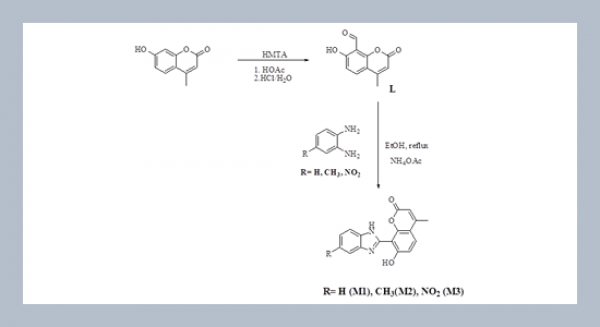
Chung Fang* Institute of Mechanics, AG3, Darmstadt University of Technology,Hochschulstr. 1, 64289 Darmstadt, Germany
Download Citation:
|
Download PDF
Goodman and Cowin proposed in 1972 a continuum theory of a dry cohesionless granular material in which the solid volume fraction n is treated as an independent kinematical field for which an additional balance law of equilibrated force is postulated. In the derivation of said balance of equilibrated of force there exists some logical inconsistency and it results in the incorrect explanation of this balance equation and the incorrect balance of internal energy. It is demonstrated that the balance of equilibrated force can be modified by a simple dimensional analysis. The resulted modified Goodman-Cowin theory is then applied to investigate the constitutive models of flowing granular materials. A complete thermodynamic analysis based upon Müller-Liu approach is performed and the constitutive responses of a granular material in thermodynamic equilibrium are obtained. From the theoretical investigations it shows that the results are more general than those obtained from the original theory and for simple shearing flow problems the current theory can reproduce all results from previous works based on the original theory. ABSTRACT
Keywords:
Goodman-Cowin theory; entropy inequality; constitutive models; granular materials.
Share this article with your colleagues
[1] Cowin, S. C. 1974. Constitutive relations that imply a generalized Mohr-Coulomb criterion. Acta Mechanica, 20: 41-46.REFERENCES
[2] Cowin, S. C. 1974. A theory for the flow of granular materials. Powder Technology, 9: 61-69.
[3] Cowin, S. C. and Goodman, M. A. 1976. A variational principle for granular materials. Zeitschrift fur Angewandte Mathematik und Mechanik, 56: 281-286.
[4] Duran, J. 2000. “Sands, Powders and Grains”. Springer Verlag New York.
[5] Fang, C. 2004. On the correspondence between the stored energy and the Helmholtz free energy functions of granular materials in thermodynamic equilibrium. Internarional Journal of Applied Science Engineering. (submitted)
[6] Goodman, M. A. and Cowin, S. C. 1972. A continuum theory for granular materials. Archive for Rational Mechanics and Analysis, 44: 249-266.
[7] Goodman, M. A. and Cowin, S. C. 1971. Two problems in the gravity flow of granular materials. Journal of Fluid Mechanics, 45: 321-339.
[8] Goodman, M. A. 1970. “A Continuum Theory for the Dynamical Behavior of Granular Materials”.D Thesis, Tulane University, U.S.A.
[9] Green, A. E. and Rivlin, R. S. 1964. Multipolar continuum mechanics. Archive for Rational Mechanics and Analysis, 17: 113-174.
[10] Hutter, K. and Rajagopal, K. R. On flows of granular materials. Continuum Mechanics and Thermodynamics, 6: 81-139.
[11] Hutter, K. 1977. The Foundations of thermodynamics, its basic postulates and implications. A review of modern thermodynamics. Acta Mechanica, 27: 1-54.
[12] Leslie, F. M. 1968. Some constitutive equations for liquid crystals. Archive for Rational Mechanics and Analysis, 28: 265-283.
[13] Liu, S. 1972. Method of langrange multipliers for exploitation of the entropy principle. Archive for Rational Mechanics and Analysis, 46: 131-148.
[14] Liu, S. 1973. On the entropy supply in a classical and a relativistic fluid. Archive for Rational Mechanics and Analysis, 50: 111-117.
[15] Mindlin, R. D. 1964. Microstructure in linear elasticity. Archive for Rational Mechanics and Analysis, 16: 51-78.
[16] Passman, S. L., Nunziato, J. W., and Walsh, E. K. 1984. A theory of multiphase mixtures. “Rational Thermodynamics”. Truesdell ed. Springer Verlag: 287-325.
[17] Savage, S. B. and Jeffrey, D. J. 1981. The stress tensor in a granular flow at high shear rates. Journal of Fluid Mechanics, 110: 255-272.
[18] Savage, S. B. 1993. Mechanics of granular flows. In: “Continuum Mechanics in Environmental Sciences and Geophysics”. K. Hutter ed. Springer Verlag: 467-522.
[19] Svendsen, B. and Hutter, K. 1995. The solid volume fraction as an internal variable for balancing evolution equation. Inernational Journal of Engineering Science, 33: 2021-2054.
[20] Toupin, R. A. 1964. Theories of elasticity with couple-stress. Archive for Rational Mechanics and Analysis, 17: 85-112.
[21] Toupin, R. A. 1962. Elastic materials with couple-stresses. Archive for Rational Mechanics and Analysis, 11: 385-414.
[22] Wang, Y. and Hutter, K. Granular material theories revisited. “Geomorphological Fluid Mechanics”. N. J. Balmforth and A. Provenzale eds. Springer Verlag: 79-107.
[23] Wang, Y. and Hutter, K. Shearing flows in a Goodman-Cowin type granular material-theory and numerical results. Particulate Science and Technology, 17: 97-124.
[24] Wilmanski, K. 1996. Using statistical orguments on the microscale. Archive for Mechanics, 48: 591-682.
ARTICLE INFORMATION
Accepted:
2003-12-23
Available Online:
2004-03-02
Fang, C. 2003. Modification of Goodman-Cowin theory and its application to the constitutive models of flowing granular materials, International Journal of Applied Science and Engineering, 2, 16–28. https://doi.org/10.6703/IJASE.2004.2.(1).16
Cite this article: